DYNAMICS

Dynamics is a branch of physical science and subdivision of mechanics that is concerned with the motion of material objects in relation to the physical factors that affect them: force, mass, momentum, energy. You might recognise this section as relating primarily to Newton's Laws of Motion.

Newton's Laws Of Motion

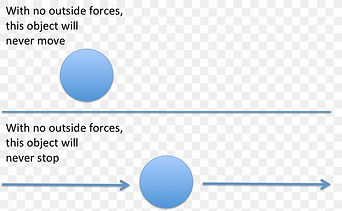
1st Law - The Law of Inertia - Objects at rest or moving at a constant velocity will tend to remain at rest or move in a constant velocity unless an external force acts upon it.

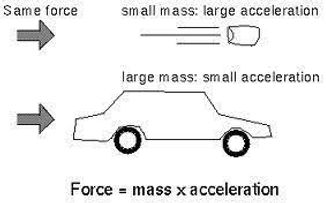
2nd Law - F = ma - The acceleration of an object depends on and is directly correlated to the mass of the object and the amount of force applied.

3rd Law - Every action has an equal and opposite reaction. In every interaction, there is a pair of forces acting on the two interacting objects. The size of the forces on the first object equals the size of the force on the second object.

System Schema + Force Diagrams

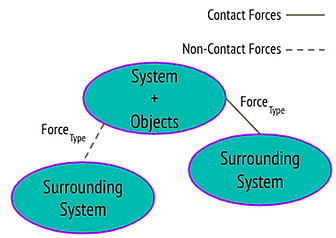
Below is an example of a System Schema:

System schema are a useful representational tool for capturing important elements about the system we are trying to describe. A system schema consists of identifying all objects of interest from a given physical situation, as well as all the different types of interactions between the objects. It basically relates an object in interest to other objects and shows directly the forces that the other objects exert on the object of interest (as shown above).

Below is an example of a force diagram:

A force diagram is a diagram showing the forces acting on the object. The object is represented by a dot with forces are drawn as arrows pointing away from the dot. In many ways the next step of the system schema, as it applies all of the information and relationships determined by the system schema. It represents the forces as vectors (which they are) and puts them into one practical, straightforward diagram.

Solving Force Problems

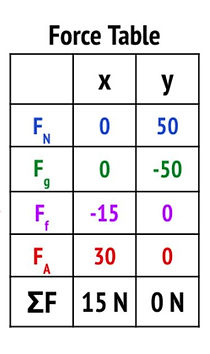
Force calculations are calculations that allow us to determine the net forces of an objects motion, this consists of finding the forces interacting on the object of interest and deriving the net force from this information by means of mathematics (mainly just adding and subtracting). System Schema and Force Diagrams could be helpful in this process, as well as a force table, which displays the forces in it's x and y components, allowing us to easily calculate vertical and horizontal net force. Pictured below is an example of one:

As the force table clearly lists out the forces in its negative and positive signs (right for left) we can easily derive the net force (∑F) by simply adding and subtracting. Some trends to notice is that the vertical (y) net force usually cancels out, as the Normal force is equal to the force of gravity Fg (most of the time).

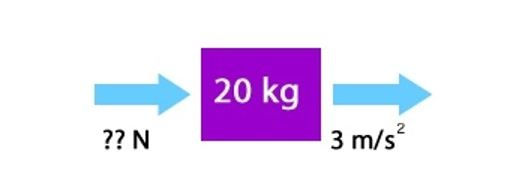
There are many other ways we could derive forces through calculation as well, Newton's Second Law would provide as a good example. Using only the mass of an object and it's acceleration, we can calculate the net force acting upon that object. For example, say I wanted to calculate the net force of a block of 20 kg in mass accelerating at 3 m/s^2:

I can simply apply Newton's Second Law (F = ma) to attain the values that I want.

*Newton's Second Law works to calculate mass and acceleration from force too. From the equation it is clear that mass = Net force/acceleration (m=F/a), and acceleration = Net force/mass (a = F/m). For example, for the problem above, mass would be 60/3 = 20 kg, and acceleration would be 60/20 = 3 m/s^2.

These are some examples of Force problems that we solved in class:

Force Calculations

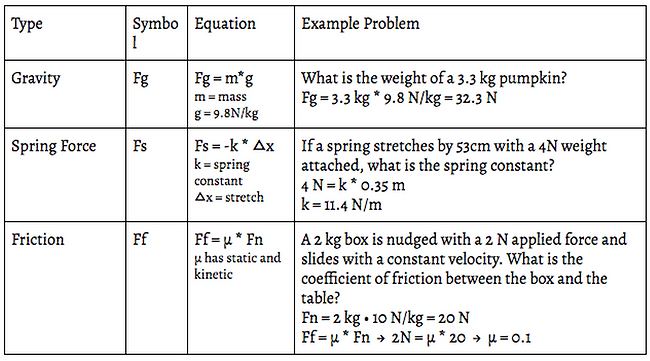
Force of Gravity

Force of gravity = Weight
Force of gravity = Mass * Gravitational Field Strength
- Fg = mg

Spring Force

Spring force is proportional to strength
Hooke's Law: Fs= -k * ∆x
- Spring Force = Spring Constant * Stretch
- Negative sign shows that the force vector and stretch vector are in opposite directions

Force of Friction

Friction Force is proportional to normal force
ƒ = µ * Fn
- Friction = Coefficient of Friction * Normal Force

Static Friction- Friction when object is not moving, changes to keep the forces balanced but has a maximum value

Kinetic Friction- Friction when object is sliding, stays constant, always less than the maximum value of the static friction

When the maximum value of the static friction is overcome, the object starts to slide

Relating Representations of Motion to Force Models

Constant velocity

Velocity = change in position / change in time
Strobe and motion maps
Position vs. time graphs (linear)
Velocity vs. time graphs (flat line)

Uniform acceleration

Acceleration = change in velocity / change in time
Velocity vs. time graphs (linear)
Acceleration vs. time graphs (flat line)
Kinematic equations

*Newton's Second Law realtes these models of motion to models of forces (force diagrams, system schemas, force tables, adding vectors, etc)

Solving Problems with Forces and Motion

Below is a video I found that I found helpful regarding this topic:

Call

123-456-7890

info@mysite.com