

ENERgy
In physics, Energy is the capacity for doing work. It exists in potential, kinetic, thermal, electrical, chemical, nuclear, or other various forms. After it has been transferred, energy is always designated according to its nature. Hence, heat transferred may become thermal energy, while work done may manifest itself in the form of mechanical energy.
Key Terms
- Energy is the quantity of matter that must be delivered to a body or physical system in order to perform work on it or heat it.
- Potential energy (U) and Kinetic energy (K) are combined to form mechanical energy (K)
- A collection of objects that we focus on is called a system
- Law of Conservation of Energy: It is possible to change the shape of energy, but it is not possible to generate or destroy it. The amount of energy in the universe remains constant.
Different types of energy include:
- Kinetic energy is the energy stored in moving things and is measured in terms of speed.
- Gravitational Potential: The amount of energy stored in the gravitational field of the Earth. The higher an object is measured from the Earth's surface, the more gravitational potential energy it has.
- Thermal/internal energy is the energy stored in a system's randomly moving atoms. Temperature is used to determine this.
- Elastic Potential is the amount of energy stored in an elastic material as a result of stretching or compressing it. The distance from equilibrium is used to calculate this.
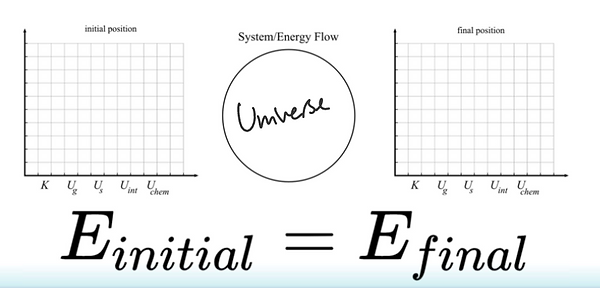
Representing Energy Transfers with LOL Charts
Below is an example of a LOL chart. The left L represents the amount of initial energies, the middle O represents the system, and the right L represents the amount of energies final.
Although energy is preserved at all times, it can be exchanged across systems in three ways: working (W), heating (Q), and radiating (R) (R). As a result, we may write Einitial + W + Q + R = Efinal as an energy conservation equation. The LOL chart can help us visualize this transition.
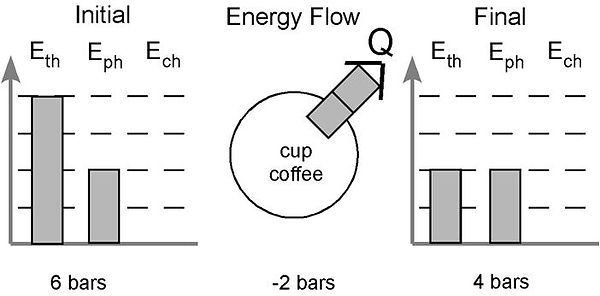
Take this example of a cup of coffee losing heat. We can clearly see that the LOL chart did a good job at representing the situation at hand. Even though the number of bars in the final chart is not as much as in the original, the LOL chart amends for this by shwong the other two bars in the system circle. This makes sense as our system was just the cup of coffee does not include the universe.
Solving Energy Problems
Before we begin to tackle a problem, lets take the following formulae into account for our problem
-
Elastic Potential energy (Us) = 1/2 kx^2 (where x is the distance from equilibrium)
-
Gravitational Potential Energy (Ug) = mgh
-
Kinetic Energy (KE) = 1/2 mv^2
Now we can go ahead and tackle the problem, consider this one, for example.
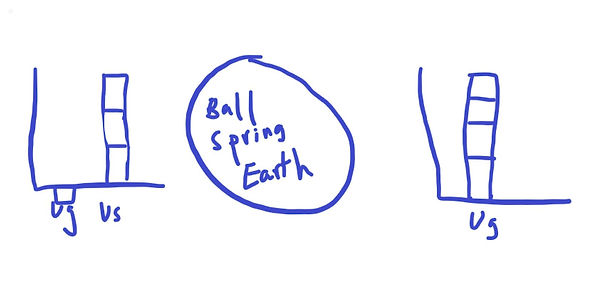
Next, we have to draw a LOL chart to represent this specific situation, this helps us to visualise the energy transfers, letting us get a better hold of the question.
We can define zero gravitational potential energy as the height of the muzzle, therefore the system starts with a negative gravitational potential energy and a lot of elastic potential energy. It's worth noting that the Earth must be included in order to have gravitational potential energy. The ball is immobile at its highest point in the ultimate position, with all of the energy stored as gravitational potential. The LOL chart gives us these calculations:
Hence the height of the ball will be 1.17 meters.

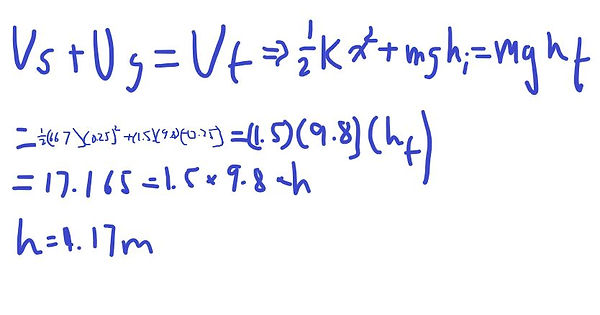
Work
Work occurs whenever a force is applied to a system, and we can detect it. It is also a transfer of energy. The formulae W = F. x or W = F. t can be used to calculate work. We take the dot product and only consider the component of the force in the direction of displacement because work is a scalar number. As a result, any forces acting perpendicular to the displacement direction have no effect on work.
Take moving a cup of coffee. When you pick it up to move it sideways, you are actually not doing work. Since the only force acting on the cup is Gravity, moving the cup sideways is perpendicular, and therefore no work is applied.
Positive work is when the work done adds energy to a system, and negative work is when work done takes away energy from the system.
Below is a video I added that does a pretty good job at explaining this topic.
Power
The rate at which work is done, is referred to as power. The equation P = W/t can be used to compute power. Watts (W) are units of measurement for power, with 1 W equaling 1 J/s. Another type of power unit you may be familiar with is horsepower (about 745 Watts). A horse can produce up to 15 horsepower, not 1, which makes you question what was going through the guy's head when he came up with this unit of measurement named after horses.
Relating Energy/Work/Power to Forces and Motion
A good example of this can be seen from doing an experiment of your personal exercises to determine how powerful you are.
The experiment requires you to complete certain tasks that allow for you to measure/ calculate your power from the measurements of force, work, reps, and distance. We did this experiment in class, performing bicep curls and stair climbs etc.
For stair climbs, we converted our weight to forces, 1 lbs = 4.45N. this yeilded a force of 647N. Since the distance was measured before hand (2.11m) and we recorded the time it took to run the stairs (1.61s), all that was left to do was to perform the calculations.
W = Fx
= (647)(2.11)
= 1392.6J
P = W/t
= 1392.6/1.61 => 894.9 watts
Another exercise that we did was bicep curls. We approached this the same way, measuring the distance (3.1m), reps (5), force (50N) and the time (3.5s). this time the force is the weight of the dumbbell, and the distance is the length of your arm multiplied by the amount of reps performed.
W = Fx
= (50)(3.1)
= 153J
P = W/t
= 153/3.5 => 44.3 watts
The terms "energy," "work," "power," "forces," and "motion" are all interchangeable terms. We saw how forces and motion are related in earlier units. Now that we understand the relationship between forces and work (W = F. x), we can see that work is a method of transferring energy, and power is the rate of this transfer.




